Genesis of Eden Diversity Encyclopedia

Get the Genesis of Eden AV-CD by secure internet order >> CLICK_HERE
Windows / Mac Compatible. Includes live video seminars, enchanting renewal songs and a thousand page illustrated codex.

Return to Genesis of Eden?

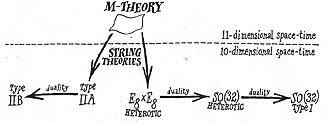
M-theory in 11 dimensions gives rise to five string theories in 10 dimensions. When the extra dimension curls into a circle, M-theory yieolds a typeIIA superstring, which is further related by a duality to the type IIB superstring. If however the extra dimension shrinks to a line segment, M-theory becomes the physically-plausible E8xE8 heterotic string. The latter is connected to the SO(32) theories by dualities.

The Theory formerly Known as Strings Michael J Duff Scientific American Feb 98

The Theory of Everything is emerging as one in which not only strings, but also membranes and black holes play a role.

At a time when certain pundits are predicting the End of Science on the grounds that all the important discoveries have already been made, it is worth emphasizing that the two niain pillars of 20th-century physics, quantum mechanics and Einstein's general theory of relativity, are mutually incompatible. General relativity fails to comply with the quantum rules that govern the behavior of elementary particles, whereas on the opposite scale, black holes are challenging the very foundations of quantum mechanics. Something big has to give. This predicament augurs less the bleak future of diminishing returns predicted by the millennial jeremiahs and more another scientific revolution. Until recently, the best hope for a theory that would unite gravity with quantum mechanics and describe all physical phenomena was based on strings: one-dimensional objects whose modes of vibration represent the elementary particles. In the past two years, however, strings have been subsumed by M-theory. In the words of the guru of string theory (and according to Life magazine, the sixth most influential American baby boomer), Edward Witten of the Institute for Advanced Study in Princeton, N.J., "M stands for Magic, Mystery or Membrane, according to taste." New evidence in favor of this theory is appearing daily, representing the most exciting development since strings first swept onto the scene. M-theory, like string theory, relies crucially on the idea of supersymmetry. Physicists divide particles into two classes, according to their inherent angular momentum, or "spin." Supersymmetry requires that for each known particle having integer spin-0, 1, 2 and so on, measured in quantum units-there is a particle with the same mass but half-integer spin ( 1/2 , 3/2, 5/2 and so on), and vice versa. Unfortunately, no such superpartner has yet been found. The symmetry, if it exists at all, must be broken, so that the postulated particles do not have the same mass as known ones but instead are too heavy to be seen in current accelerators. Even so, theorists have retained belief in supersymmetry primarily because it provides a framework within which the weak, electromagnetic and strong forces may be united with the most elusive force of all: gravity. Supersymmetry transforms the coordinates of space and time such that the laws of physics are the same for all observers. Einstein's general theory of relativity derives from this condition, and so supersymmetry implies gravity. In fact, supersymmetry predicts "supergravity," in which a particle with a spin of 2-the graviton-transmits gravitational interactions and has as a partner a gravitino, with a spin of 3/2. Conventional gravity does not place any limits on the possible dimensions of space-time: its equations can, in principle, be formulated in any dimension. Not so with supergravity, which places an upper limit of 11 on the dimensions of space-time. The familiar universe, of course, has three dimensions of space: height, length and breadth, while time makes up the fourth dimension of spacetime. But in the early 1920s Polish physicist Theodore Kaliiza and Swedish physicist Oskar Klein suggested that spacetime may have a hidden fifth dimension. This extra dimension would not be infinite, like the others; instead it would close in on itself, forming a circle. Around that circle could reside quantum waves, fitting neatly into a loop. Only integer numbers of waves can fit around the circle; each of these wotild correspond to a particle with a different energy. So the energies would be "quantized," or discrete. An observer living in the other four dimensions, however, would see a set of particles with discrete charges, rather than energies. The quantum, or unit, of charge would depend on the circle's radius. In the real world as well, electrical charge is quantized, in units of e, the charge on the electron. To get the right value for e, the circle would have to be tiny, about 10^-33 centimeter in radius. The unseen dimension's small size explains why humans, or even atoms, are unaware of it. Even so, it would yield electromagnetism, and gravity, already present in the four-dimensional world, would be united with that force. In 1978 Eugene Creamer, Bernard Julia and Joel Scherk of the tcole Normale Sup6rieure in Paris realized that supergravity not only permits up to seven extra dimensions but is most elegant when existing in a space-time of 11 dimensions (10 of space and one of time). The kind of real, four-dimensional world the theory ultimately predicts depends on how the extra dimensions are rolled up, a la Kaluza and Klein. The several curled dimensions could conceivably allow physicists to derive, in addition to electromagnetism, the strong and weak nuclear forces. For these reasons, many physicists began to look to supergravity in 11 dimensions in the hope that it might be the unified theory. In 1984, however, 11-dimensional supergravity was rudely knocked off its pedestal. An important feature of the real world is that nature distinguishes between right and left: the laws governing the weak nuclear force operate differently when viewed through a mirror. (For instance, neutrinos always have left-handed spin.) But as Witten and others emphasized, such "handedness" cannot readily be derived by reducing space-time from 11 dimensions down to four.

P-Branes

Supergravity's position was usurped by superstring theory in 10 dimensions. Five competing theories held sway, designated by their mathematical characteristics as the E8 x E8 heterotic, the SO(32) heterotic, the SO(32) Type 1, the Type IIA and Type IIB strings. (The Type I is an "open" string consisting of just a segment, whereas the others are 14 closed" strings that form loops.) One string in particular, the E8 x E8, seemed at least in principle@apable of explaining the known elementary particles and forces, including their handedness. And unlike supergravity, strings seemed to provide a theory of gravity consistent with quantum effects. All these virtues enabled string theory to sweep physicists off their feet and 11-dimensional supergravity into the dog house. Murray Gell-Mann of the California Institute of Technology encapsulated the mood of the times by declaring at a meeting: "Eleven-dimensional supergravity-ugh!' After the initial euphoria over strings, however, nagging doubts began to creep in. First, many important questions especially how to confront the theory with experiment-seemed incapable of being answered by traditional methods of calculation. They called for radically new techniques. Second, why were there five different string theories? If one is looking for a unique Theory of Everything, surely this is an embarrassment of riches. Third, if supersymmetry permits 1 1 dimensions, why do superstrings stop at 10? Finally, if we are going to conceive of pointlike particles as strings, why not as membranes or more generally as p-dimensional objects-inevitably dubbed p-branes? Consequently, while most theorists were tucking into super-spaghetti, a small but enthusiastic group were developing an appetite for super-ravioli. A particle, which has zero dimensions, sweeps out a one-dimensional trace, or 44 worldline," as it evolves in space-time [see illustration on next page]. Similarly a string-having one dimension, lengthsweeps out a two-dimensional "worldsheet," and a membrane-having two dimensions, length and breadth-sweeps out a three-dimensional '4worldvolume. " In general, a p-brane sweeps out a worldvolume of p+1 dimensions. (Of course, there must be enough room for the pbrane to move about in space-time, so p+1 must not exceed the number of space-time dimensions.) As early as 1962, Paul A. M. Dirac, one of the fathers of quantum mechanics, had constructed an imaginative model based on a membrane. He postulated that the electron, instead of resembling a point, was in reality a minute bubble, a membrane closed in on itself. Its oscillations, Dirac suggested, might generate particles such as the muon, a heavier version of the electron. Although his attempt failed, tlie equations that Dirac postulated for the membrane are esselitially tlie ones we use today. The membrane may take tlie fortil of a bubble, or it may be stretched out in two directions like a sheet of rubber. Supersymmetry severely restricts the possible dimensions of a P-brane. In the space-time of 11 dimensions floats a membrane, discovered mathematically by Eric Bergshoeff of the University of Groningen, Ergin Sezgin, now at Texas A&M University, and Paul K. Townsend of the University of Cambridge. It has only two spatial dimensions and looks like a sheet. Paul S. Howe of King's College London, Takeo Inami of Kyoto University, Kellogg Stelle of Imperial College, London, and I were able to show that if one of the 11 dimensions is a circle, we can wrap the membrane around it once, pasting the edges together to form a tube. If the radius of the circle becomes sufficiently small, the rolledup membrane ends up looking like a string in 10 dimensions; in fact, it yields precisely the Type IIA superstring. Notwithstanding such results, the membrane enterprise was largely ignored by the orthodox string community. Fortunately, the situation was about to change because of progress in an apparently unrelated field. In 1917 German mathematician Amalie Emmy Noether had shown that the mass, charge and other attributes of elementary particles are generally conserved because of symmetries. For instance, conservation of energy follows if one assumes that the laws of physics remain unchanged with time, or are symmetric as time passes. And conservation of electrical charge follows from a symmetry of a particle's wave function. Sometimes, however, attributes may be maintained because of deformations in fields. Such conservation laws are called topological, because topology is that branch of mathematics that concerns itself with the shape of things. Thus, it may happen that a knot in a set of field lines, called a soliton, cannot be smoothed out. As a result, the soliton is prevented from dissipating and behaves much like a particle. A classic example is a magnetic nionopole-the isolated pole of a bar magnet-which has not been found in nature but shows up as twisted configurations in some field theories. In the traditional view, then, particles such as electrons and quarks (which carry Noether charges) are seen as fundamental, whereas particles such as magnetic monopoles (which carry topological charge) are derivative. In 1977, however, Claus Montonen, now at the Heisinki Institute of Physics, and David 1. Olive, now at the University of Wales at Swansea, made a bold conjecture. Might there exist an alternative formulation of physics in which the roles of Noether charges (like electrical charge) and topological charges (like magnetic charge) are reversed?

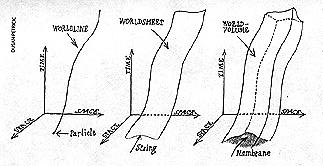
TRAJECTORY of a particle in spacetime traces a worldline. Similarly, that of a string or a membrane sweeps out a worldsheet or worldvolume, respectively.

In such a "dual" picture, the magnetic monopoles would be the elementary objects, whereas the familiar particles-quarks, electrons and so on-would arise as solitons. More precisely, a fundamental particle with charge e would be equivalent to a solitonic particle with charge 1/e. Because its charge is a measure of how strongly a particle interacts, a monopole would interact weakly when the original particle interacts strongly (that is, when e is large), and vice versa. The conjecture, if true, would lead to a profound mathematical simplification. In the theory of quarks, for instance, physicists can make hardly any calculations when the quarks interact strongly. But any monopoles in the theory must then interact weakly. One could imagine doing calculations with a dual theory based on monopoles and automatically getting all the answers for quarks, because the dual theory would yield the same final results. Unfortunately, the idea remained on the back burner. It was a chicken-andegg problem. Once proved, the Montonen-Olive conjecture could leap beyond conventional calculational techniques, but it would need to be proved by some other method in the first place. As it turns out, p-branes can also be viewed as solitons. In 1990 Andrew Strominger of the Institute for Theoretical Physics in Santa Barbara found that a 1 0-dimensional string can yield a soliton that is a five-brane. Reviving an earlier conjecture of mine, Strominger suggested that a strongly interacting string is the dual equivalent of weakly interacting five-branes. There were two major impediments to this duality. First, the duality proposed by Montonen and Olive-between electricity and magnetism in ordinary four dimensions-was still unproved, so duality between strings and five-branes in 10 dimensions was even more tenuous.

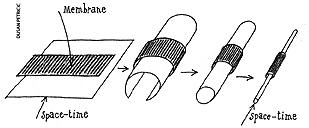
SIMULTANEOUS SHRINKING of a membrane and a dimension of space-time can result in a string. As the underlying space, shown herc as a two-dimcnsional shcet, curls into a cylinder, the membrane wraps around it. The curled dimension bccomcs a circle so small that the two-dimensional space ends up looking one-dimensional, like a line. The tightly wrapped membrane then resembles a string.

Second, there were all kinds of issues about how to find the quantum properties of five-branes and hence how to prove the new duality. The first of these impediments was removed, however, when Ashoke Sen of the Tata Institute of Fundamental Research in Bombay established that SLIpersymmetric theories would require the existence of certain solitons with both electrical and magnetic charges. These objects had been predicted by the Montonen-Olive conjecture. This seemingly inconspicuous result converted many skeptics and unleashed a flood of papers. In particular, it inspired Nathan Seiberg of Rutgers University and Edward Witten to look for duality in more realistic (though still supersymmetric) versions of quark theories. They provided a wealth of information on quantum fields, of a kind unthinkable just a few years ago.

Duality of Dualities

In 1990 several theorists generalized the idea of Montonen-Olive duality to four-dimensional superstrings, in whose realm the idea becomes even more natural. This duality, which nonetheless remained speculative, goes by the name of S-duality. In fact, string theorists had already become used to a totally different kind of duality called T-duality. T-duality relates two kinds of particles that arise when a string loops around a compact dimension. One kind (call them "vibrating" particles) is analogous to those predicted by Kaluza and Klein and comes from vibrations of the loop of string [see illustration on next page]. Such particles are more energetic if the circle is small. In addition, the string can wind many times around the circle, a rubber band on a wrist; its energy becomes higher the more times it wraps around and the larger the circle. Moreover, each energy level represents a new particle (call them "winding" particles). T-duality states that the winding particles for a circle of radius R are the same as the "vibration" particles for a circle of radius 1/R, and vice versa. To a physicist, the two sets of particles are indistinguishable: a fat, compact dimension may yield apparently the same particles as a thin one. This duality has a profound implication. For decades, physicists have been struggling to understand nature at the extremely small scales near the Planck length of 10^-33 centimeter. We have always supposed that laws of nature, as we know them, break down at smaller distances. What T-duality suggests, however, is that at these scales, the universe looks just the same as it does at large scales. One may even imagine that if the universe were to shrink to less than the Planck length, it would transform into a dual universe that grows bigger as the original one collapses. Duality between strings and fivebranes still remained conjectural, however, because of the problem of quantizing five-branes. Starting in 1991, a team at Texas A&M, involving jianxin Lu, Ruben Minasian, Ramzi Khuri and myself, solved the problem by sidestepping it. If four of the 10 dimensions curl up and the five-brane wraps around these, the latter ends up as a one-dimensional object-a (solitonic) string in six-dimensional space-time. In addition, a fundamental string in 10 dimensions remains fundamental even in six dimensions.

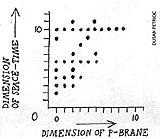"BRANE" SCAN lists the membranes that arise in space-times of different dimensions. A p-brane of dimension 0 is a particle, that of dimension 1 is a string and that of dimension 2 is a sheet or bubble. Some branes have no spin (red), but Dirichiet-branes have spin of 1 (blue).

So the concept of duality between strings and five-branes gave way to another conjecture, duality between a solitonic and a fundamental string. The advantage is that we do know how to quantize a string. Hence, the predictions of string-string duality could be put to the test. One can show, for instance, that the strength with which the solitonic strings interact is given by the inverse of the fundamental string's interaction strength, in complete agreement with the conjecture. In 1994 Christopher M. Hull of Queen Mary and Westfield College, along with Townsend, suggested that a weakly interacting heterotic string can even be the dual of a strongly interacting Type IIA string, if both are in six dimensions. The barriers between the different string theories were beginning to crumble. it occurred to me that string-string duality has another unexpected payoff. If we reduce the six-dimensional spacetime to four dinietisions, by curling lip two dimensions, the fundamental string and the solitonic string each acquire a T-dLiality. But here is the niiracle: the T-duality of the solitonic string is just tile S-duality of the fundamental string, and vice versa. This phenomenon-in which the interchange of charges in one picture is just the inversion of length in the dual picture-is called the Duality of Dualities. It places the previously speculative S-duality on just as firm a footing as the well-established T-duality. In addition, it predicts that the strength with which objects interact-their charges-is related to the size of the invisible dimensions. What is charge in one universe may be size in another.

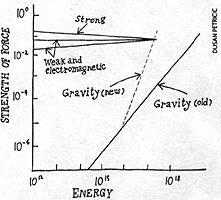THREE FORCES CONVERGE to the same strength when particles are as energetic as 10 ^16 giga electron volts. Until now, gravity was believed to miss this meeting point. But calculations including the llth dimension of M-theory suggest that gravity as well may converge to the same point.

In a landmark talk at the University of Southern California in 1995, Witten suddenly drew together all the work on T-duality, S-duality and string-string duality under the umbrella of M-tiieory in 1 1 dimensions. In the following months, literally hundreds of papers appeared on the Internet confirming that whatever M-theory may be, it certainly involves membranes in an important way. Even the E8 x E8 string, whose handedness was thought impossible to derive from 11 dimensions, acquired an origin in M-theory. Witten, along with Petr Horava of Priiiceton University, showed how to shrink the extra dimension of M-theory into a segment of a line. The resulting picture has two 10-dimensionai universes (each at ati end of the line) coiiiiected by a space-time of 11 dimensions. Particles-and strings-exist only in the parallel universes at the ends, which can coiiimuiiicate with each other only via gravity. (One can speculate that all visible matter in our universe lies oil one wall, whereas the "dark matter," believed to account for the invisible mass iii the universe, resides in a parallel universe on the other wall.) This scenario may have important consequences for confronting M-theory with experiiiieiit. For example, physicists know that the intrinsic strengths of all the forces change with the energy of the relevant particles. In supersymmetric theories, one finds that the strengths of the strong, weak and electromagnetic forces all converge at an energy E of 10^16 giga electron volts. Further, the interaction strengths almost equal-but not quite-the value of the dimensionless number GE^2, where G is Newton's gravitational constant. This near miss, most likely not a coincidence, seems to call for an explanation; it has been a source of great frustration for physicists. But in the bizarre space-time envisioned by Horava and Witten, one can choose the size of the 11 th dimension so that all four forces meet at this common scale. It is far less than the Planck energy of 10^19 giga electron volts, at which gravity was formerly expected to become strong. (High energy is connected to small distance via quantum mechanics. So Planck energy is simply Planck length expressed as energy.) So quantum-gravitational effects may be far closer in energy to everyday events than physicists previously believed, a result that would have all kinds of cosmological consequences.

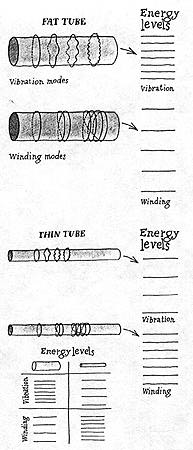Duality between large and small

T-duality connects the physics of large space-times with that of small ones. Visualize a curled space-time as a cylinder. A string looped around it has two kinds of energy states. One set arises from the waves in the string-that fit around the cylinder; call these the 'vibration' modes. If the cylinder is fat, the vibrations tend to have long wavelengths and less energy. So the energies corresponding to different numbers of waves around the cylinder are separated by small amounts-that is, they are "closely spaced.' The string can, however, also loop around the cylinder like a stretched rubber band. If the cylinder is fat, the string needs to stretch more, requiring more energy. So the energies of the states corre sponding to different numbers of loops-call these the'winding' modes-are widely spaced. Now look at the energy levels for a thin cylin der. The waves fitting around it are small and so have high energy. As a result, the vibration states are widely spaced. But the loops re quire less energy, and so the winding modes are closely spaced. To an outside observer, however, the different physical origins of the vibration and winding states are not apparent. Both the thin and the fat tube yield ulti mately the same energy levels, which physicists interpret as particles. Thus, the minute scales of the thin space time may yield exactly the same physics as the large scales of our . verse. MJ. D.

Recently Joseph Poichinski of the Institute for Theoretical Physics at Santa Barbara realized that some p-branes resemble a surface discovered by 19thcentury German mathematician Peter G. L. Dirichiet. On occasion these branes can be interpreted as black holes or, rather, black-branes-objects from which nothing, not even light, can escape. Open strings, for instance, may be regarded as closed strings, part of which are hidden behind the black-branes. Such breakthroughs have led to a new interpretation of black holes as intersecting black-branes wrapped around seven curled dimensions. As a result, there are strong hints that M-theory may even clear up the paradoxes of black holes raised by Stephen W Hawking of the University of Cambridge. In 1974 Hawking showed that black holes are in fact not entirely black but may radiate energy. In that case, black holes must possess entropy, which measures the disorder of a system by accounting for the number of quantum states available. Yet the microscopic origin of these quantum states stayed a mystery. The technology of Dirichlet-branes has now enabled Strominger and Cumrun Vafa of Harvard University to count the number of quantum states in blackbranes. They find an entropy that agrees perfectly with Hawking's prediction, placing another feather in the cap of M-theory. Black-branes also promise to solve one of the biggest problems of string theory: there seem to be billions of different ways of crunching 10 dimensions down to four. So there are many competing predictions of how the real world works-in other words, no prediction at all. It turns out, however, that the mass of a black-brane can vanish as a hole it wraps around shrinks. This feature miraculously affects the space-time itself, allowing one space-time with a certain number of internal holes (resembling a Gruy&re cheese) to change to another with a different number of holes, violating the laws of classical topology. If all the space-times are thus related, finding the right one becomes a more tractable problem. The string may ultimately choose the space-time with, say, the lowest energy and inhabit it. Its undulations would then give rise to the elementary particles and forces as we know them-that is, the real world.

10 to 11: Not Too Late

Despite all these successes, physicists are glimpsing only small comers of M-theory; the big picture is still lacking. Recently Thomas Banks and Stephen H. Shenker of Rutgers University, together with Willy Fischier of the University of Texas and Leonard Susskind of Stanford University, have proposed a rigorous definition of M-theory. Their "matrix" theory is based on an infinite number of zero-branes (particles, that is). The coordinates, or positions, of these particles, instead of being ordinary numbers, are matrices that do not commute-that is, xy does not equal yx. In this picture space-time itself is a fuzzy concept in which the coordinates cannot be defined as the usual numbers but instead as matrices. Physicists have long suspected that unifying gravity-in other words, the geometry of space-time-with quantum physics will lead to space-time becoming similarly ill defined-at least until a new definition is discovered. The matrix approach has generated great excitement but does not yet seem to be the last word. Over the next few years, we hope to discover what M-theory really is. Witten is fond of imagining how physics might develop on another planet, where major discoveries such as general relativity, quantum mechanics and supersymmetry are made in a different order than on Earth. In a similar vein, I would like to suggest that on planets more logical than ours, 11 dimensions would have been the starting point from which 10-dimensional string theory was subsequently derived. Indeed, future terrestrial historians may judge the late 20th century as a time when theorists were like children playing on the seashore, diverting themselves with the smoother pebbles or prettier shells of superstrings while the great ocean of M-theory lay undiscovered before them.